Create art using math
Imagine you’re in a clothing store full of many different patterned coats. Which patterns do you most easily recognize? Polka dot, stripes, houndstooth, argyle, plaid? What about any tessellation patterns? Tessellation may be a big word, but it’s really just the manipulation of polygons into a pattern with no gaps or overlaps.

There are three main types of tessellations
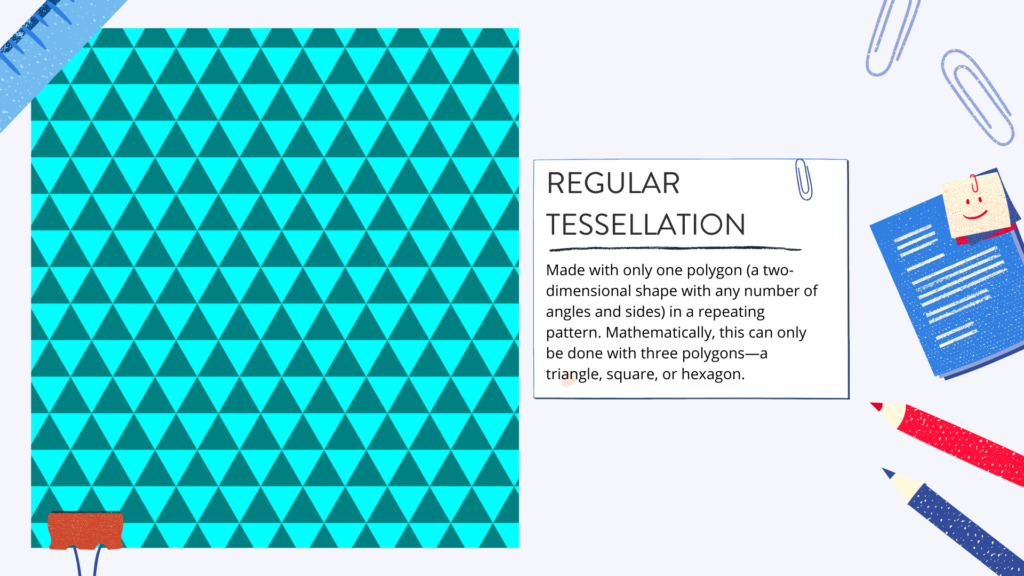
- A regular tessellation is made with only one polygon (a two-dimensional shape with any number of angles and sides) in a repeating pattern. Mathematically, this can only be done with three polygons—a triangle, square, or hexagon.
- A semi-regular tessellation is made with two or more polygons, and there are only eight variations that exist. Using triangles and squares and hexagons creates additional polygons in the negative space, like octagons and dodecagons!

- A non-regular tessellation can be made with any shape, as long as the pattern repeats. These are where you can get really creative, using made-up shapes with all sorts of edges and borders and squiggles.

Make a tessellation
Let’s start by making a Regular Tessellation, which is made with only one polygon in an interlocking, repeating pattern.
- Draw and cut out the polygon of your choice from a thick sheet of paper.
- Using the shape you cut out as your template, trace the polygon.
- While there is no wrong way to arrange your polygon, remember that the main characteristic of a tessellation is the repetition of the shape without gaps or overlaps!
- Make sure to turn and flip your shape in order to leave no gaps. Your triangles may end up upside down, but they’re still triangles!
- Once your paper is filled with the shape you traced, do you see a pattern? Can you see the repetition?
- Use colors to fill in the shapes. You should notice your tessellated pattern, so be conscious of your color choices to make the pattern stand out even more!
Once you’ve created your Regular Tessellation, cut out a few different polygons to try your hand at a semi-regular tessellation. If you’re feeling extra bold and creative, try the non-regular tessellation! Remember: with a non-regular tessellation, you can use any polygon from your imagination, as long as you can fit it together interlockingly with no gaps or overlaps. This is a fun mathematical brain challenge and all you need is scissors, paper, colors, and your brain!
Continue Learning
- Just like a bee’s honeycomb, there are naturally-occurring tessellations in nature. Can you think of any other examples?
- Use the same technique but mix up your colors and shapes and sizes!
- Do an image search for artwork by M. C. Escher, a Dutch artist who frequently used mathematical themes in his art. Using the glossaries from the book, can you find examples of math concepts in his artwork, like geometric, polygon, and translational symmetry?
The source for all of this great information is an eBook available through Hoopla, Cool Tessellations by Anders Hanson and Elissa Mann. Check it out and keep the learning and fun going with a glossary of terms, beautiful tessellation examples, and increasingly challenging projects, like making tessellations in 3-D!
Sources
- Merriam-Webster Dictionary
- Cool Tessellations eBook by Anders Hanson and Elissa Mann.
200 SE Martin Luther King Jr. Blvd
Evansville, Indiana 47713
Administration: ceo@evpl.org
Card & Account: circulation@evpl.org
Feedback & Ideas: marketing@evpl.org



